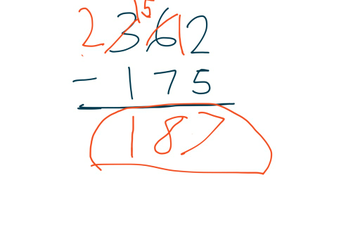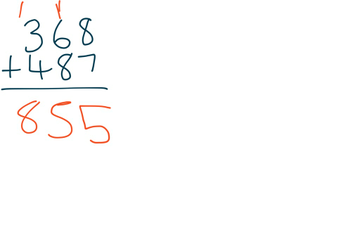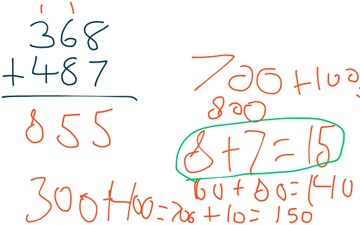Number Talks
Developing accuracy, flexibility & efficiency in computation
Overview
Originally, this collaborative inquiry sought to investigate the impact on student learning of using problem-based learning in mathematics. This approach promotes the use of the mathematical processes, and makes student thinking visible. Research suggests that learning in this context deepens conceptual understanding. As the inquiry progressed, it became apparent that many students had not developed a deeper conceptual understanding of addition and subtraction by participating in a series of 3 part, problem-based lessons. Furthermore, the research conducted demonstrated that many students were over-reliant on the use of the standard algorithm when working on addition and subtraction questions, and had limited flexibility in the use of alternative strategies. To address these issues, the teachers involved decided to integrate Number Talks into their math programs as a way to promote flexibility, accuracy and efficiency in computation, and to help students to consolidate their understanding of the big ideas of quantity relationships, counting and operational sense.
Context
This collaborative inquiry was conducted as part of the Ontario Ministry of Education's Student Work Study (SWS) Initiative. A SWS inquiry is centred around a theory of action developed by the participating Student Work Study Teacher (SWST), and host classroom teachers (in this case two grade 4 teachers). It involves an iterative cycle of co-planning, co-teaching, and co-debriefing, with the SWST acting as a researcher to document student thinking and learning. To learn more about Collaborative Inquiry in Ontario please click the link below.
The school where this inquiry took place is a large K-5 school in Brampton, Ontario. This school has a high proportion of Canadian-born English Language Learners.
Phase One
The focus for the original theory of action for this inquiry was developed after I (SWST) shared my initial observations of student thinking with the host teachers, and the host teachers shared their student's strengths and areas of need. Both the host teachers and I felt that the majority of students appeared to demonstrate considerable procedural proficiency in mathematics. The evidence discussed indicated that the majority of students were able to:
-represent, compare and order whole numbers,
-demonstrate an understanding of place value by representing them in expanded form
-round numbers to the nearest ten, hundred and thousand
-count forwards and backwards by various numbers
The host teachers also noted that some students had memorized many of the basic facts for addition, subtraction, multiplication and division, and had some familiarity with the standard algorithms for addition and subtraction. They expressed some concern that much of this procedural proficiency had been learned by rote, without developing the complimentary conceptual understanding. The host teachers expressed an interested in integrating problem-based learning into their mathematics programs. As such we developed the following theory of action for our inquiry:
'If we use a problem-based learning approach to math that fosters the use of accountable talk, then the students will develop their oral, visual, and written communication to make their thinking visible. This, in turn, will help the students to develop deeper conceptual understanding.'
Why use a problem-based learning approach to mathematics?
- problem solving is the primary focus and goal of mathematics in the real world
- to help students develop deeper conceptual understanding (Small, 2013 pg. 3)
- it is consistent with constructivist and socio-cultural theories of learning (Van de Walle et al, 2014)
- research supports its implementation, see, for example, Carpenter & Lehrer (1999), Ross, Hogaboam-Gray & McDougall (2002), Boaler & Staples (2008)
- to foster positive attitudes towards mathematics and problem solving
- to promote the use of the mathematical processes
- to develop independence
- it promotes perseverance and confidence
- it allows for the use of open questions and parallel tasks to differentiate instruction
- it makes student thinking visible, and, as such, gives the teacher insight into student understanding and misconceptions, providing teachers with information from which they can plan future instruction
First problem-based lesson
Effective collaboration and dialogue are essential to the success of a 3-part, problem-based math lesson. To help foster accountable talk we engaged the students in a 'Looks like, sounds like, feels like' activity prior to the lesson. They were asked to think about what the classroom would look, sound, and feel like if students were working together successfully to solve a math problem. See the picture below for student responses. We also introduced the students to a 'Talk Prompts' anchor chart as the lesson began to scaffold the use of accountable talk.
The question used for the first lesson came from Marian Small's Good Questions - Great Ways to Differentiate Mathematics Instruction:
'A number describes how many students are in a school. What might the number be?'
Some samples of the student solutions are pictured below.
The students participated in a 'Gallery Walk' part way through the lesson as a way to begin to build shared understanding. The host teachers then facilitated a 'Math Congress' at the end of the lesson to highlight certain strategies, and different ways of thinking. Please click the following link to the Ministry Monograph entitled 'Communication in the Mathematics Classroom' for a description of these instructional approaches.
Reflections at the end of Phase One
As the lesson was co-debriefed (by reviewing my field notes and examining student products) the following points were noted:
- students were engaged and persevered for long periods
- some students experienced difficulty collaborating with their partner
- as we had not chosen a specific learning goal, the lesson lacked focus
- only a small number of students engaged meaningfully in the math congress
- some students engaged in the gallery walk, others were unfocussed
- student thinking became visible and the teachers were able to gather formative assessment data
Next steps
In keeping with the iterative nature of this inquiry the following next steps were developed for phase two:
1. Develop clear learning goals for future lessons (based on curriculum expectations).
2. Assign different partners to encourage collaboration.
3. Develop accountability during the Gallery Walk by using the 'one stay, one stray' protocol.
4. Continue to promote the use of 'Talk Prompts', and encourage more student to student interaction during subsequent math congresses.
5. Annotate student work during the math congress to make ideas clear for all students.
6. Summarize the key learnings at the end of each lesson.
Phase 2
For phase two of the inquiry we planned a series of 3-part, problem-based lessons on addition and subtraction.
As we co-planned this series of lessons we used our 'next steps' from the end of phase one as a guide. We chose an overall curriculum expectation, and then developed a clear learning goal that would allow us to focus the consolidation part of each lesson on a few key ideas.
The curriculum expectation we chose was:
By the end of grade 4 students will:
'Solve problems involving the addition and subtraction of four digit numbers, using student generated algorithms (and standard algorithms)'
The learning goal we developed was:
'To gain a conceptual (vs. procedural) understanding of addition and subtraction and how it relates to place value'
One of our main aims for these lessons was to encourage the use of alternative algorithms. The section below called 'What the research says' details the justification for encouraging the use of alternative algorithms over the standard algorithm.
We predicted that the vast majority of students would attempt to use the standard algorithm, and so we told the students that they must use other strategies to solve the lesson problem.
What the research says
Issues associated with the use of the standard algorithm
- Standard algorithms proceed from left to right (from the units), and so students lose a sense of the quantity of the whole numbers involved (Fosnot & Dolk, 2001 pg 122).
- Students find them difficult to use because they have to switch their thinking between performing the addition or subtraction and regrouping (Small, 2013).
- A study by Kamii & Dominick (1998) showed that algorithms can be harmful to children's mathematical development, and that the range of wrong answers was much bigger for students who had been taught and used the algorithm, i.e. there is a much higher occurrence of unreasonable answers.
Why encourage alternative algorithms?
- Some research studies have shown that students show greater achievement when they develop their own algorithms (Hiebert, 1984; Kamii, Lewis & Livingston (1993).
- Students develop their number sense and their facility to compose and decompose numbers (Small, 2013; Fosnot & Dolk, 2001).
- They are easier to use mentally.
- They are often more efficient.
- Different algorithms/strategies work better for different numbers.
- Students typically make fewer errors (Van de Walle et al, 2013) possibly because these strategies are more meaningful as students have constructed them themselves (Small, 2013).
Co-planning the lessons using the 5 Practices
We decided to create a series of three lessons; one on addition, one on subtraction, and one with a multi-step problem that would require students to use both operations. Once we developed the questions, we decided to use Smith and Stein's (2011) 5 Practices framework to plan the lessons.
The following picture provides an overview of the 5 Practices.
Having completed the 'Anticipating' phase of the 5 Practices by solving the problem ourselves, and by consulting the Guide to Effective Instruction in Mathematics to identify the strategies the students might use we created the following charts to assist us in the 'Monitoring' phase of the lesson.
Monitoring chart for lesson 1 (addition)
Monitoring chart for lesson 2 (subtraction)
Monitoring chart for lesson 3 (addition & subtraction)
Below are some pictures of some of the strategies that students used to solve the question in lesson one of phase two. The question was:
'Three hundred forty two people went to Wonderland on Friday. Four hundred ninety-five people went on Saturday. How many people went to Wonderland on these two days?
Below are some pictures of the strategies that students used to solve the problem in lesson two of phase two. The question was:
'The largest gorilla has a mass of about two hundred seventy-five kilograms. The largest orangutan has a mass of one hundred twenty-seven kilograms. What is the difference in their masses?'
Regrouping using base ten blocks
Regrouping using expanded form
Partial subtraction
Compensation & Constant Difference
Compensation & Constant Difference
Compensation & Constant Difference
Below are some pictures of the strategies that students used to solve the problem in lesson three of phase two. The question was:
'Ms. C. and Ms. S. went on a trip to Quebec City. Google maps tells them that Quebec City is 837km away from Brampton. They want to check out the different sites of interest along the way so it takes them three days to get there. On the first day they drove 249km. On day two they travelled 336km. How far do they have to travel on day three to reach Quebec City?'
Adding on & right handed subtraction (both with errors)
Regrouping using base ten blocks (subtraction)
Regrouping using expanded form (addition) and base ten blocks (subtraction)
Regrouping using base ten blocks
Regrouping using base ten blocks & adding on
Regrouping using expanded form & adding on
Splitting & Adding On
Splitting & Adding On
Partial Subtraction
Moving & Partial Subtraction
Moving & Partial Subtraction
Compensation
Assessment
At the end of phase 2 three different assessments were administered to determine if students had achieved the learning goal we developed for this series of lessons:
'To gain a conceptual (vs. procedural) understanding of addition and subtraction and how it relates to place value'
We also wanted to see if the students would independently use some of the strategies they had been introduced to during phase 2.
Assessment 1
The first assessment was a paper and pencil task where all students in both classes were asked to answer one addition and one subtraction question (very similar to the lesson problems) using any strategy they liked. The questions used were:
1. Tim Horton's served four hundred forty-three customers on Tuesday and three hundred ninety-five customers on Wednesday. How many customers did Tim Horton's serve on these two days? Show your work.
2. The average caribou has a mass of about two hundred sixty-five kilograms. The average moose has a mass of four hundred thirty kilograms. What is the difference in their masses? Show your work.
Results
The analyses of this assessment are pictured below. As is clear from these tables, the vast majority of the students used the standard algorithm for both questions.
Points of note:
- only 6 students attempted to use one of the strategies (splitting) introduced in the series of three lessons for the addition question - no students used adding on, moving or compensation for this question
- for the subtraction question one student used the adding on strategy, and three used a variant of the splitting strategy used for addition - no students used partial subtraction, compensation or constant difference for this question
- 8 students added the numbers instead of subtracting for question 2
- 88% of students answered the addition question correctly, with all of the students using the standard algorithm computing correctly
- 52% of students answered the subtraction correctly, with 9 students using the standard algorithm incorrectly (of 31 that used it)
Assessment 2
Of the students that used the standard algorithm for assessment 1, and used it correctly, 21 were asked to explain how the algorithm works. Their explanations were recorded using the app Educreations.
Results
The recordings were analyzed for appropriate use of place value language (e.g., 'I regrouped one hundred in the tens column which became ten tens' or 'I added three hundred and seven hundred') as the students described how they use the algorithm
- 16 of the 21 students recorded used no place value language at all (see recording of student S below)
- 4 of the 21 students used some place value language e.g. talked about adding/subtracting the ones/tens/hundreds column (see recording of student H below)
- 1 of the 21 students used appropriate place value language consistently in his description e.g. talked about adding the ones/tens/hundreds column, and talked about adding three hundred plus four hundred (see recording of student B below)
Assessment 3
A representative sample of students were asked to solve two addition and two subtraction questions mentally. They then explained the strategy they used, and this was recorded by the SWST using the app Explain Everything.
The questions for this assessment used two digit numbers. The students involved in this inquiry were in grade 4. By the end of grade 3 students are expected to be able to add and subtract two digit numbers mentally. The numbers used for each question were specifically chosen to encourage the use of different strategies.
The sample consisted of 17 students in total of which about half typically achieve levels 1 and 2, and the other half achieving levels 3 and 4, in math, as measured by the classroom teachers summative assessments, and the provincial report card.
Results
- Students used a mental 'regrouping' strategy (a right handed strategy based on the standard algorithm) about 60% of the time.
- Students used alternative (left handed) strategies such as splitting and moving 14% of the time.
- The level 1 and 2 students used the alternative strategies less than 1% of the time.
- The level 3 and 4 students used the alternative strategies 24% of the time.
- Certain strategies were not used at all. These included adding on (for addition), moving, compensation (for subtraction), partial subtraction and constant difference.
- Students used a strategy that could not be identified or named (one based on a misconception that led to an incorrect answer) 26% of the time.
- The level 1 and 2 students did this about 47% of the time, whereas the level 3 and 4 students did this less than 1% of the time.
The short videos below show a representative sample of student responses.
Reflections at the end of Phase Two
As we co-debriefed these lessons (by reviewing the SWSTs field notes, examining student products, watching videos of the consolidation part of each lesson, and examining the assessment results) the following points were noted:
- asking students to use alternative algorithms promoted significant amounts of thinking
- many students showed considerable perseverance
- some students showed frustration at not just being allowed to use the standard algorithm
- students working through level 2 required a significant amount of guidance before being able to begin to solve the problem
- we were unable to find an open-ended question to address our learning goal
- it was difficult to choose numbers for the problems that would encourage a variety of strategies
- these lessons required a significant time commitment (two double periods for each of the three lessons)
- the teachers had to engage in significant amounts of prompting and questioning to elicit the use of alternative strategies, very few students generated alternative algoritms independently
- the majority of students used right-handed methods similar to the standard algorithm with a heavy reliance on base-ten blocks, and the use of expanded form
- the few students that used alternative algorithms had received explicit instruction in their use in previous grades
- allowing significant time for students to work, and asking probing questions, made student thinking more visible, this surfaced misconceptions that could be addressed in the moment
- some students began to show some evidence of conceptual understanding of regrouping as they modeled their strategy with base ten blocks
- some students continued to experience challenges working with their peers
- some students viewed the gallery walk as 'cheating'
- during the math congress we began to see some emergence of student to student interaction, and some sporadic use of the talk prompts
- some growth in the students ability to explain their thinking both visually, and orally was evident
Next steps
Based on these reflections we decided on the following next steps for phase 3.
1. Continue pursuing a problem-based learning approach to math in other strands to promote the use of the math processes
2. Try an alternative instructional approach to address the fact that many students:
-are over-reliant on the standard algorithm and right handed methods for addition and subtraction
-have not consolidated their understanding of place value and the principles of numeration
Resources Used in Phases 1 and 2
5 Practices
Young Mathematicians at Work
Good Questions
Making Math Meaningful
Math Expressions
Teaching Student-Centred Mathematics
Phase Three
Having consulted our next steps from phase 2, we decided that phase 3 of our inquiry would focus on developing an instructional approach that would promote the use of alternative strategies for computation, and help students to consolidate their understanding of place value and number sense.
Marian Small states that:
'The idea is not to 'present' any [algorithms] at the start, but to allow students to invent their own. Many algorithms will come up with minimal prompting' (Small, 2013, pg. 219)
However, our experiences from phase 2 seemed at odds with this statement. We had provided considerable prompting, and very few student-invented algorithms had emerged. Even after students had been introduced to these alternative algorithms a number of times the vast majority were not using them independently.
Small goes on to say that:
'It is not wrong for students to continue to use their own algorithms. If the algorithms are inefficient, students are likely to figure that out for themselves' (Small, 2013 pg. 218)
Again, our experiences seemed to contradict this statement. We found that students will persist with inefficient methods (e.g. using models such as base ten blocks) even after they have been introduced to more efficient strategies.
As we read further, Fosnot & Dolk (2001) also seemed to contradict Small. They have found that children are most likely to invent 'splitting' strategies, but will rarely, if ever, construct more powerful strategies like adding on and constant different. They talk about the fact that we need to start with children's own constructions, but then need to help children develop a repertoire of strategies that focus on the patterns and relationships in number.
We had started with children's own constructions. Now we needed a more guided instructional approach that would help students to compute with number sense. We decided that we would use the resource Number Talks which seemed to offer the approach we were looking for.
New Theory of Action
We developed the following theory of action to guide our work in phase 3 of the inquiry:
'If we use Number Talks on a regular basis, as part of a balanced math program, then the students will be able to compute numbers with more accuracy, efficiency and flexibility whilst also displaying a greater sense of number and understanding of place value.'
What are Number Talks?
from the resource
"A five- to fifteen-minute classroom conversation around purposefully crafted computation problems that are solved mentally." (Parrish, 2014)
Number Talks are designed to help students develop a repertoire of computation strategies (many of which can be used mentally) that they can use flexibly, efficiently and accurately. They build upon foundational mathematical concepts of the base-ten system, and the composition and decomposition of numbers. They help to elicit strategies that focus on the relationships between numbers. As students participate in a Number Talk they share and defend their strategy which gives them the opportunity to practice their use of the math processes of communicating and reasoning.
In the following video Professor Jo Boaler provides an overview of Number Talks.
The following video shows a Number Talk on subtraction from a grade 3 classroom.
Co-planning
As we began to co-plan the Number Talks lesson we decided to begin with some basic strategies using one and digit numbers such as 'making tens', 'making landmark numbers' and 'doubles and near doubles'. We had observed many students, especially those working through level two, using their fingers to compute with small numbers. These first Number Talks that we planned aimed to address this issue and help students to compute more efficiently.
Key Learnings
- It is essential for students to have developed a solid conceptual understanding of the big ideas in numeration to be able to compute effectively, especially using mental math.
- Standard algorithms present significant barriers to children's mathematical development.
- The use of alternative algorithms helps children develop into flexible mathematical thinkers rather than students who follow rules without understanding the underlying concepts.
- Students are very reliant on standard algorithms, and their use is so ingrained that they are unlikely to generate their own alternative algorithms without a significant amount of guidance.
- When students struggle to compute numbers effectively they experience heavy 'cognitive load' when solving math problems.
References
Boaler, J., & Staples, M. (2008) Creating mathematical futures through and equitable teaching approach: The case of Railside School. Teachers' College Record. 110 (3), 608-645.
Carpenter, T.P., & Lehrer, R. (1999) Teaching and learning mathematics with understanding. In Fennema, E., & Romberg, T.A. (Eds) Mathematics classrooms that promote understanding. Mahwah, NJ; Lawrence Erlbaum Associates, 19-32
Fosnot, C.T., & Dolk, M. (2001) Young Mathematicians at Work: Constructing Number Sense, Addition, and Subtraction. Portsmouth, NH; Heinemann
Hiebert, J. (1984) Children's mathematics learning: The struggle to link form and understanding. Elementary School Journal, 84, 496-513.
Kamii, C., & Dominick, A. (1999) The harmful effects of algorithms in grades 1-4. In Morrow, L, & Kenney, M.J. (Eds) The Teaching and Learning of Algorithms in School Mathematics. Reston, VA; NCTM, 130-140.
Kamii, C., Lewis, B.A. & Livingston, S.J. (1993) Primary arithmetic: Children inventing their own procedures. Arithmetic Teacher, 41, 200-203.
Ontario Ministry of Education (2006) A Guide to Effective Instruction in Mathematics, Kindergarten to Grade 6: Volume Two - Problem Solving & Communication. Toronto, ON; Queen's Printer for Ontario.
Parrish, S. (2014) Number Talks: Helping Children Build Mental Math & Computation Strategies. Sausolito, CA; Math Solutions.
Ross, J. A., Hogaboam-Gray, A., & McDougall, D. (2002) Research on reform in mathematics education, 1993-2000. Alberta Journal of Educational Research, 48, 122-138.
Small, M. (2013) Making Math Meaningful to Canadian Students, K-8. Toronto, ON; Nelson.
Smith, M.S., & Stein, M.K. (2011) 5 Practices for Orchestrating Productive Mathematics Discussions. Reston, VA; NCTM.
Van de Walle, J.A. et al (2014) Teaching Student-Centred Mathematics: Developmentally Appropriate Instruction for Grades 3-5. Toronto, ON; Pearson